Geometry

Geometry is the study of figures, which are technically called manifolds.
Differential geometry is the field of the precise study of the shape of manifolds, using cues such as curvature and the behaviour of geodesics of (Riemannian) manifolds. Here geodesics are generalisations of straight lines in Euclidean space. On the other hand, topology is the field of the study of topological properties of manifolds by mapping algebraic invariants onto them.
The basic algebraic invariants, for instance, are fundamental groups, homotopy groups, homology groups and cohomology groups.

	Prof. KONDO Kei E-mail: keikondo@(okayama-u.ac.jp) Global Riemannian geometry (especially geodesic theory), Non-smooth analysis, Exotic structures, Minimal submanifolds from aspects of PDEs, and Origami Directory of Researchers　Researchmap

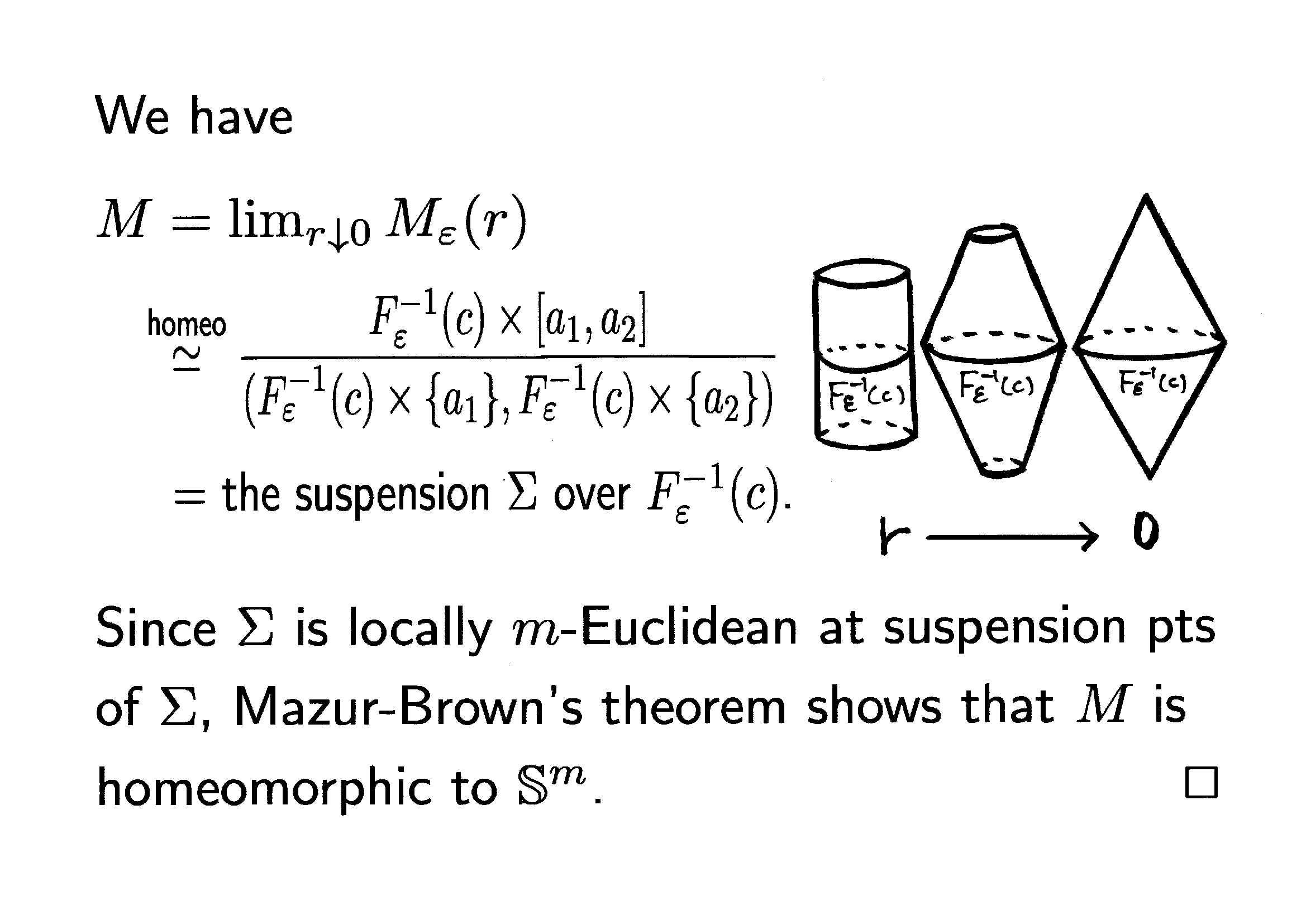
I have studied the relationship between curvature and topology of Riemannian and Finsler manifolds using geodesic theory, and have also studied exotic spheres and origami through non-smooth analysis. In recent years my interest has shifted to minimal submanifolds (geometric analysis) from a PDE perspective.

American Mathematical Society; Mathematical Reviews